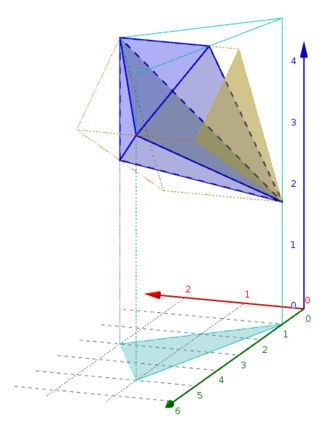
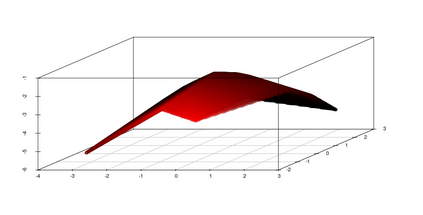
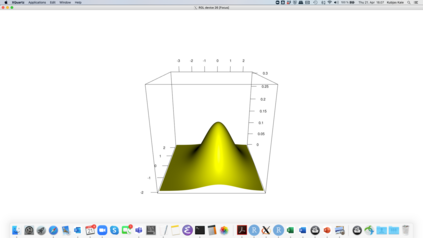
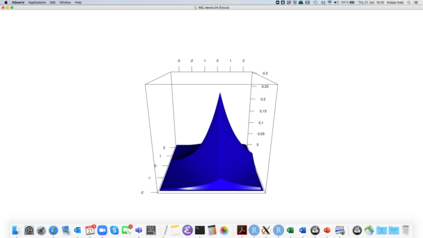
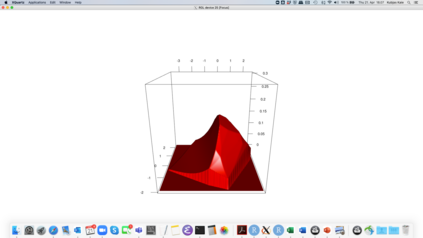
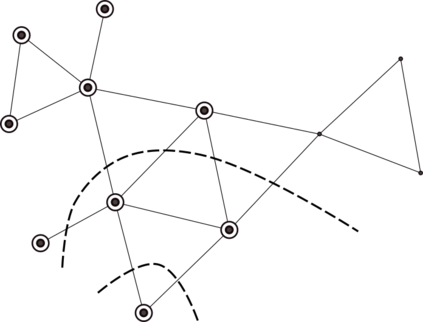
We study the problem of maximum likelihood estimation of densities that are log-concave and lie in the graphical model corresponding to a given undirected graph $G$. We show that the maximum likelihood estimate (MLE) is the product of the exponentials of several tent functions, one for each maximal clique of $G$. While the set of log-concave densities in a graphical model is infinite-dimensional, our results imply that the MLE can be found by solving a finite-dimensional convex optimization problem. We provide an implementation and a few examples. Furthermore, we show that the MLE exists and is unique with probability 1 as long as the number of sample points is larger than the size of the largest clique of $G$ when $G$ is chordal. We show that the MLE is consistent when the graph $G$ is a disjoint union of cliques. Finally, we discuss the conditions under which a log-concave density in the graphical model of $G$ has a log-concave factorization according to $G$.
翻译:我们研究了对正辛醇-腐蚀性密度的最大可能性估计问题,这些问题存在于与某一非方向图形相对应的图形模型中。我们显示,最大可能性估计(MLE)是几个帐篷函数的指数的产物,每个最大类别为$G美元,一个最大类别为$G美元。虽然图形模型中的对正辛醇-腐蚀性密度是无限的,但我们的结果表明,通过解决一个有限维度的锥形优化问题,可以找到最大可能性估计密度。我们提供了执行和几个例子。此外,我们表明,只要抽样点大于最大类别($G美元为chordal)的大小,MLE就存在最大可能性估计(MLE),而且概率1是独一无二的。我们表明,当图形“$G”是粘结的时,MLE是一致的。最后,我们讨论了图形模型($G美元)的正辛醇密度根据$G$G$的日对日汇系数进行日算系数化的条件。