Geometric analysis of gaits and optimal control for three-link kinematic swimmers

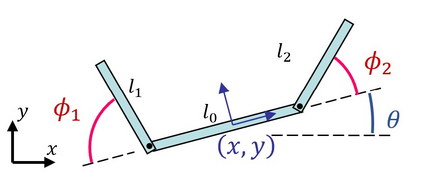
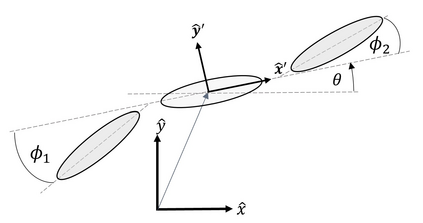
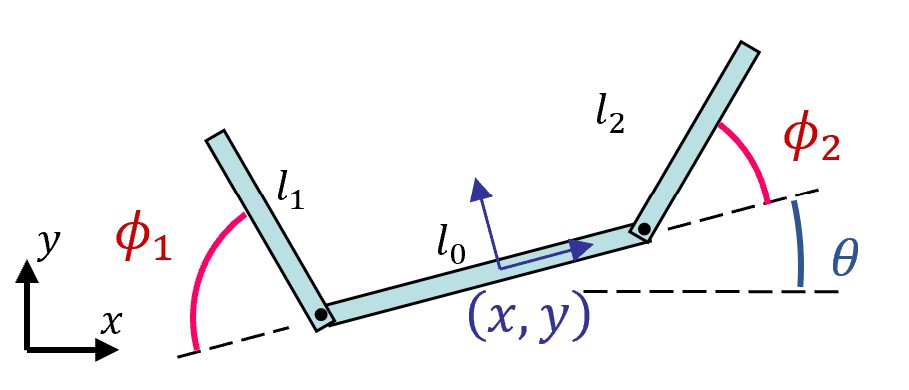
Many robotic systems locomote using gaits - periodic changes of internal shape, whose mechanical interaction with the robot's environment generate characteristic net displacements. Prominent examples with two shape variables are the low Reynolds number 3-link "Purcell swimmer" with inputs of 2 joint angles and the "ideal fluid" swimmer. Gait analysis of these systems allows for intelligent decisions to be made about the swimmer's locomotive properties, increasing the potential for robotic autonomy. In this work, we present comparative analysis of gait optimization using two different methods. The first method is variational approach of "Pontryagin's maximum principle" (PMP) from optimal control theory. We apply PMP for several variants of 3-link swimmers, with and without incorporation of bounds on joint angles. The second method is differential-geometric analysis of the gaits based on curvature (total Lie bracket) of the local connection for 3-link swimmers. Using optimized body-motion coordinates, contour plots of the curvature in shape space give visualization that enables identifying distance-optimal gaits as zero level sets. Combining and comparing results of the two methods enables better understanding of changes in existence, shape and topology of distance-optimal gait trajectories, depending on the swimmers' parameters.