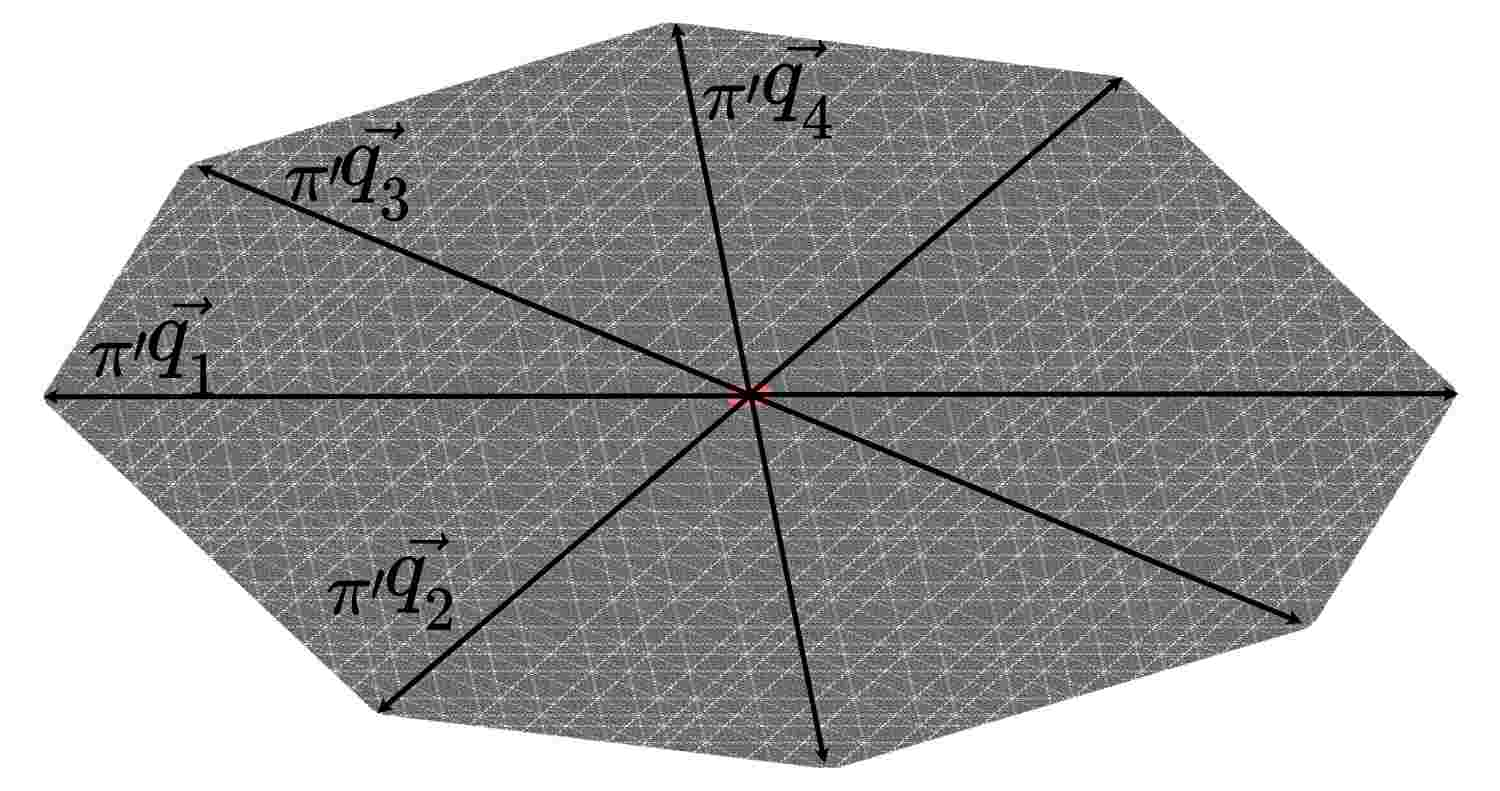
Self-assembly is the process in which the components of a system, whether molecules, polymers, or macroscopic particles, are organized into ordered structures as a result of local interactions between the components themselves, without exterior guidance. In this paper, we speak about the self-assembly of aperiodic tilings. Aperiodic tilings serve as a mathematical model for quasicrystals - crystals that do not have any translational symmetry. Because of the specific atomic arrangement of these crystals, the question of how they grow remains open. In this paper, we state the theorem regarding purely local and deterministic growth of Golden-Octagonal tilings. Showing, contrary to the popular belief, that local growth of aperiodic tilings is possible.
翻译:自我组装的过程是一个系统的各个组成部分,无论是分子、聚合物还是宏观粒子,在没有外部指导的情况下,由于部件本身之间的局部相互作用而组织成有条结构的过程。 在本文中,我们谈论的是周期性砖块的自我组装。周期性砖块作为准晶体的数学模型,这些晶体没有任何翻译性对称。由于这些晶体的特殊原子安排,它们是如何生长的问题仍然开放的。我们在本文中陈述了纯本地和决定性地增长的金-奥克坦形砖块的理论。 与流行的信念相反,它表明周期性砖块的局部增长是可能的。