A $J$-Symmetric Quasi-Newton Method for Minimax Problems

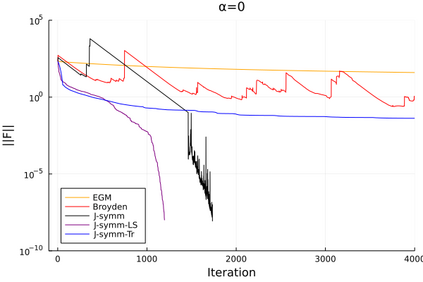
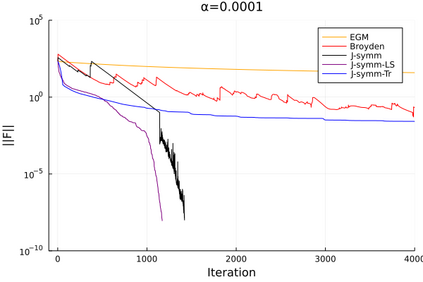
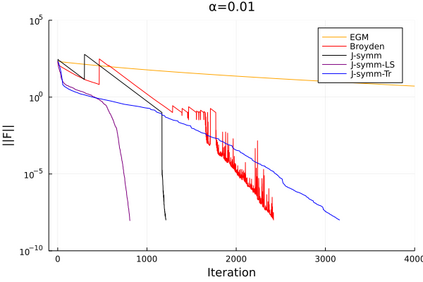
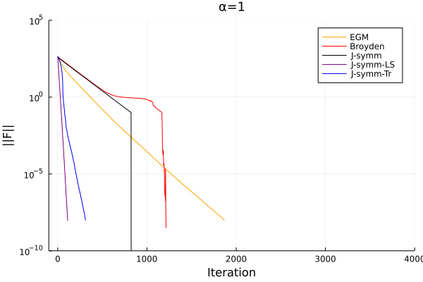
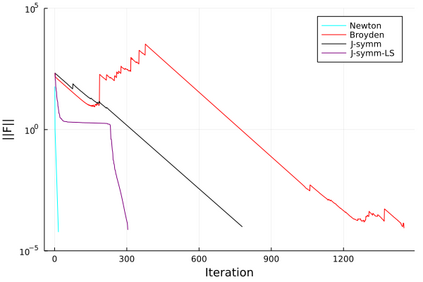
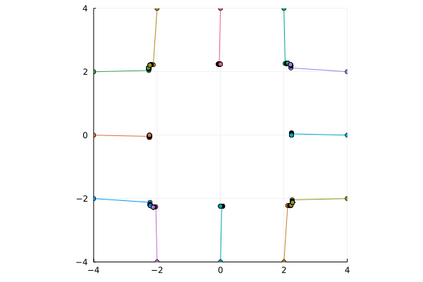
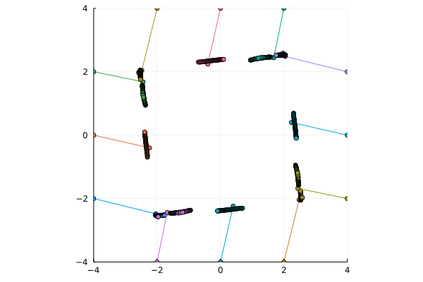
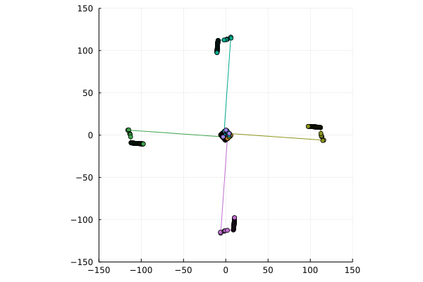
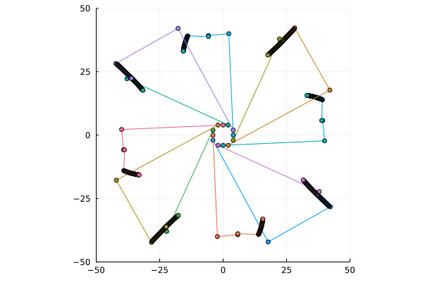
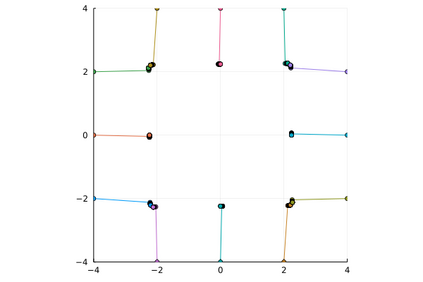
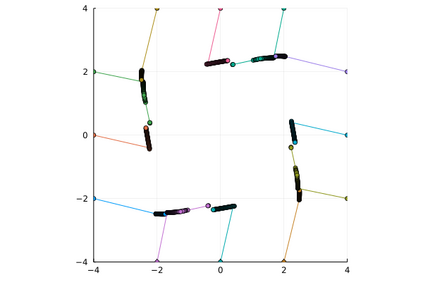
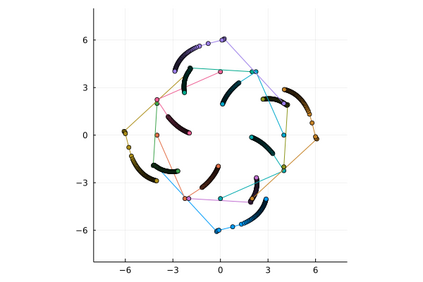
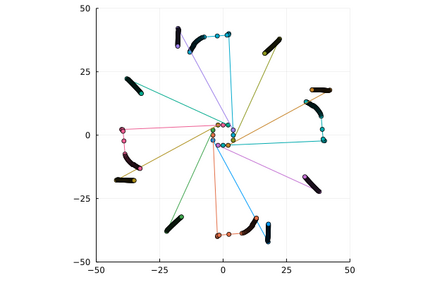
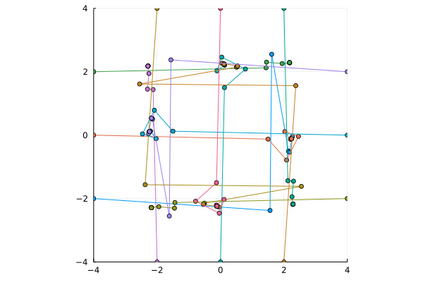
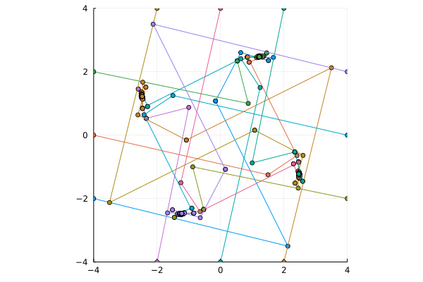
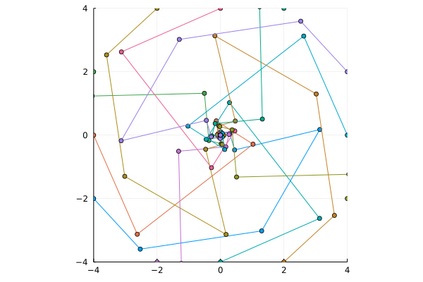
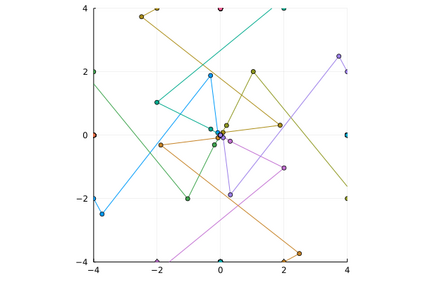
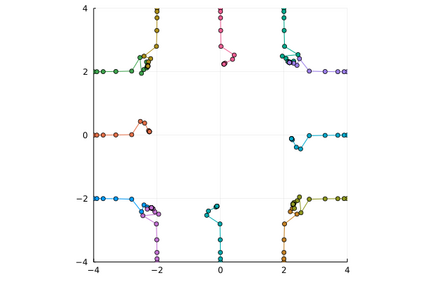
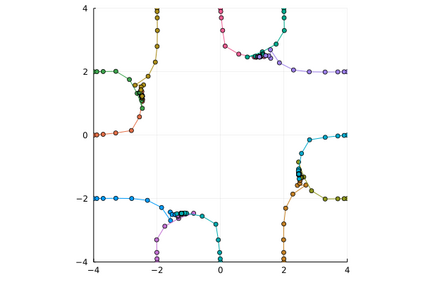
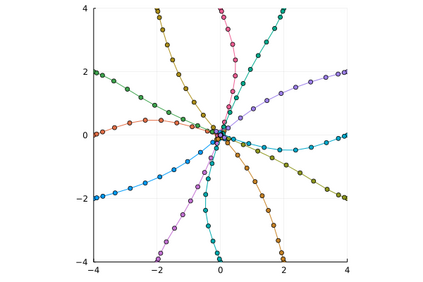
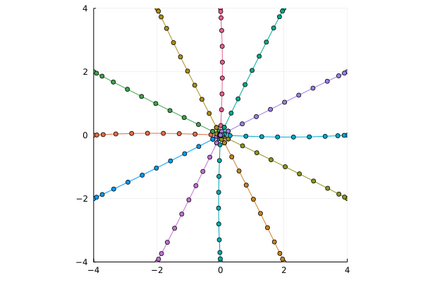
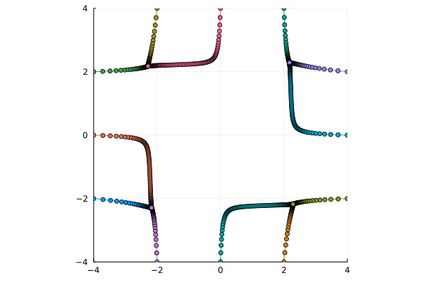
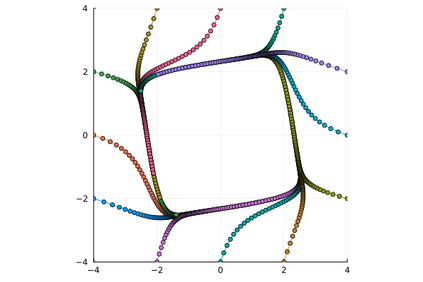
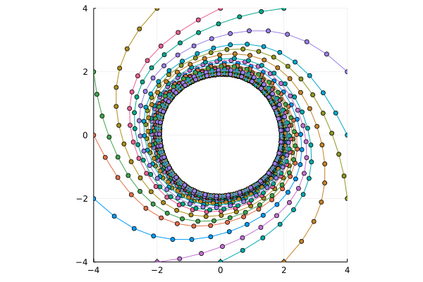
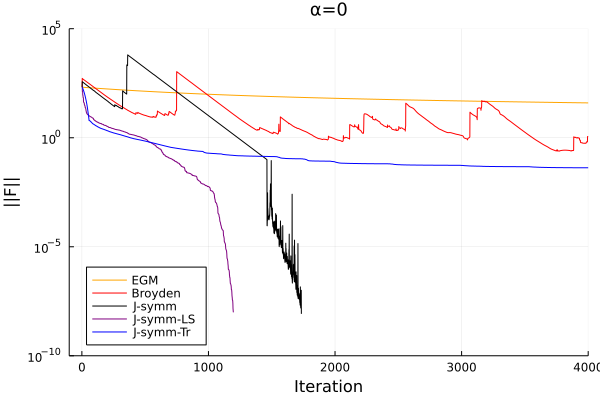
Minimax problems have gained tremendous attentions across the optimization and machine learning community recently. In this paper, we introduce a new quasi-Newton method for minimax problems, which we call $J$-symmetric quasi-Newton method. The method is obtained by exploiting the $J$-symmetric structure of the second-order derivative of the objective function in minimax problem. We show that the Hessian estimation (as well as its inverse) can be updated by a rank-2 operation, and it turns out that the update rule is a natural generalization of the classic Powell symmetric Broyden (PSB) method from minimization problems to minimax problems. In theory, we show that our proposed quasi-Newton algorithm enjoys local Q-superlinear convergence to a desirable solution under standard regularity conditions. Furthermore, we introduce a trust-region variant of the algorithm that enjoys global R-superlinear convergence. Finally, we present numerical experiments that verify our theory and show the effectiveness of our proposed algorithms compared to Broyden's method and the extragradient method on three classes of minimax problems.